 b 1 US" width="479" height="479" />
b 1 US" width="479" height="479" />Here you will learn about the slope-intercept form of a line, y=m x+b, including how to recognize the slope and y -intercept from the equation y=m x+b and rearrange an equation into slope intercept form y=m x+b.
Students first work with linear equations in 7 th grade when they learn about the constant of proportionality and direct variation. They expand their knowledge as they move through 8 th grade and high school math.
y=m x+b is the slope intercept form of the line. There are other ways to write the equation of a straight line like standard form or point slope form. However, the focus here is going to be on the slope-intercept form of the equation of a line, y=m x+b.
y=m x+b is a linear equation because when it’s graphed on the coordinate plane, it forms a line. You can also determine this because the power of x is equal to 1.
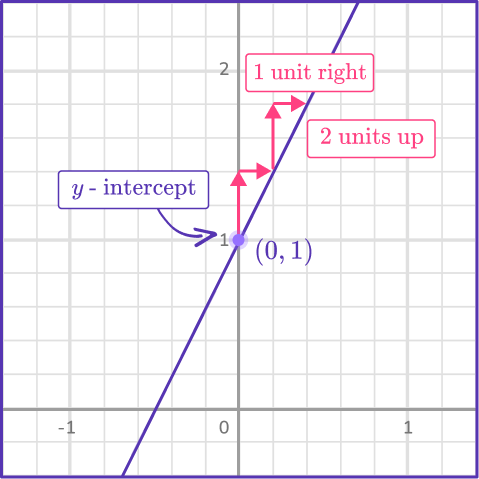
Let’s take a look at the linear equation y=2 x+1.
Since m is represented by the number 2 and b is represented by the number 1. You can state that the slope of this line is 2 and the y -intercept is 1.
Graphically, you can see that the ordered pair of the y -intercept is (0, 1) and the slope is represented by 2 units up and 1 unit to the right.
 b 1 US" width="479" height="479" />
b 1 US" width="479" height="479" />
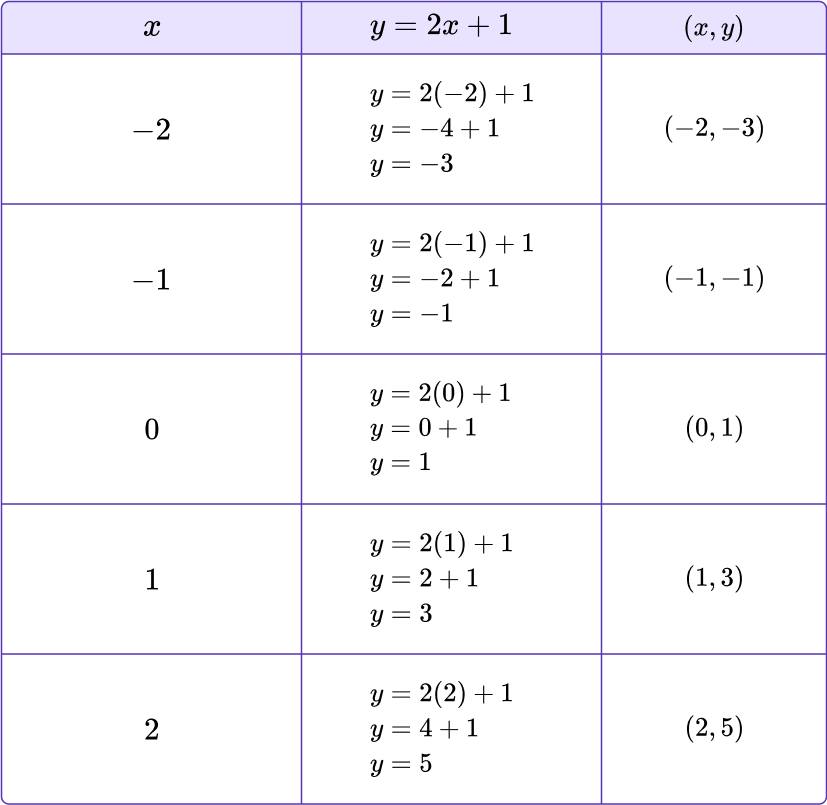
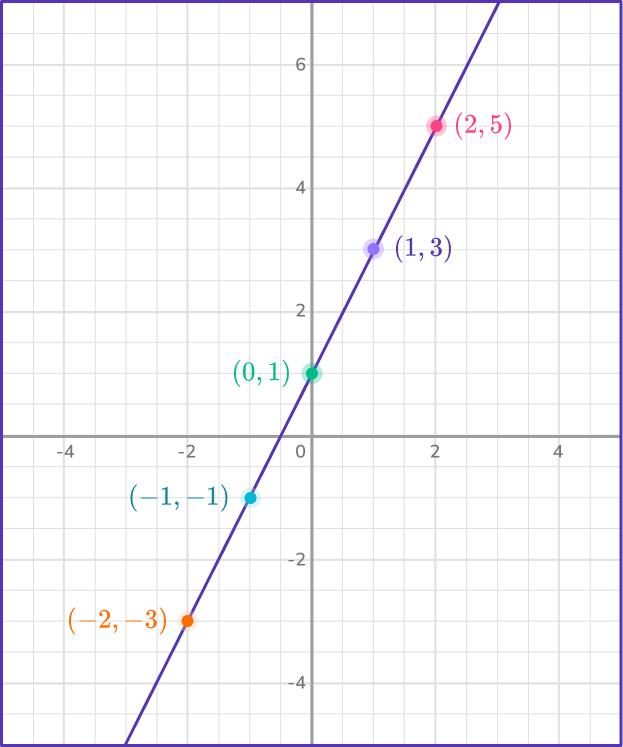
Since the x and y represent the coordinates of any point on the line, you can create a table of values to find ordered pairs to represent the line graphically. Recall that the independent variable is x and the dependent variable is y.
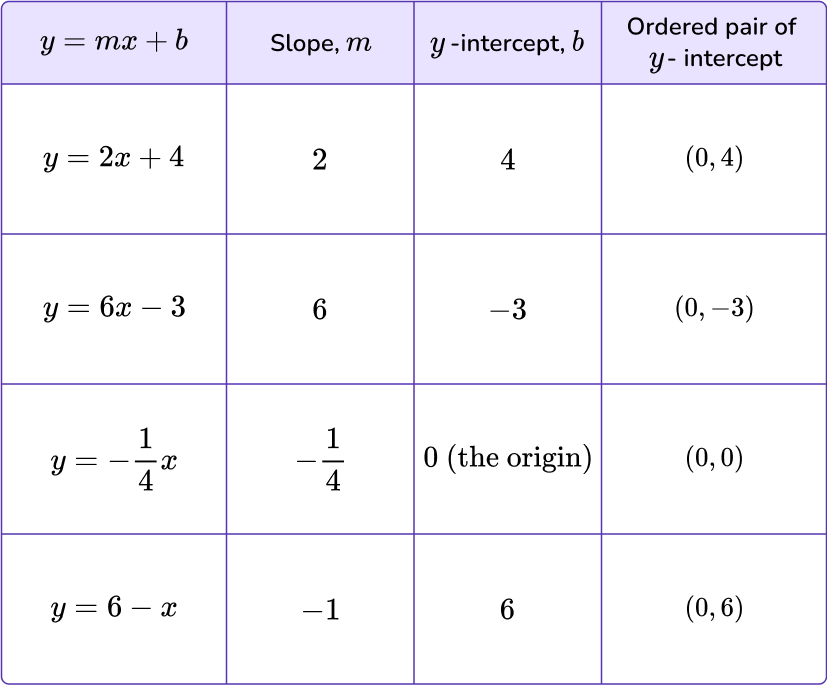
Here is a quick summary of some equations in the form y=m x+b with the slope and y intercept highlighted.
Special cases:
Horizontal lines
Horizontal lines have a slope of 0.
So, the equation in slope-intercept form of the horizontal line below can be expressed as, y=0 x+3 which can be written as y=3.

Vertical lines:
Vertical lines have an undefined slope.
So, the equation of the line graphed below can be expressed as x=3. The equation of all vertical lines will be the x value of where the line intersects the x axis.